I personally usually stick with option 1, simply because its easier

But I would still contend that option 2 is viable. As mentioned, empirical results (i.e., actual live gameplay) is nicely modeled by option 2.
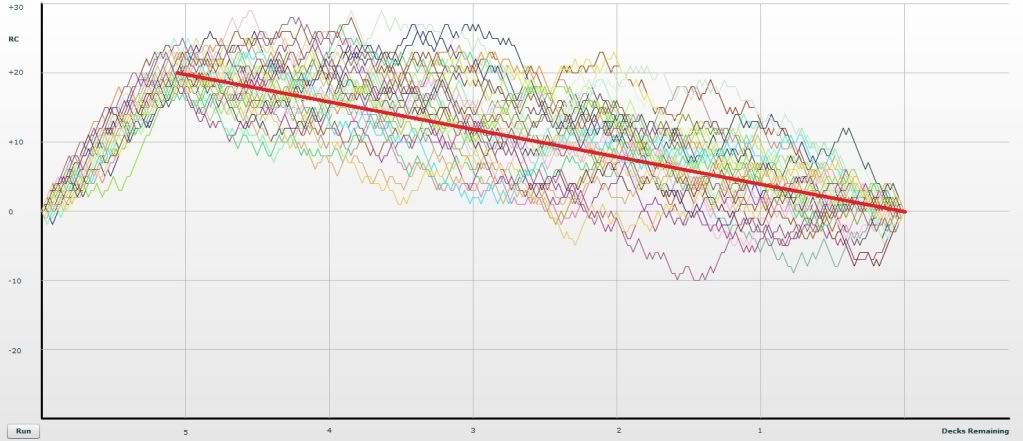
For example, lets say very early in the shoe, after 1 deck of play, the RC is extremely high, e.g., +20. At this point what do we expect from experience? Invariably we would expect this RC to go down (gravitate towards 0, and I'm not necessarily saying going to 0) as we continue further into the shoe. Sure, it might go up for a while, then go down, or go down below a 0 count, and shoot back up, or gradually go down to +10 etc., but typically we expect, and it does go down.
We would not expect the RC to hover around +20 or trend upwards, and it rarely would. The few times this has happened to me, I'm usually taken aback, the deck seems out of whack, and I suspect I might have lost the count (and probably have).
I think Option 2 models this well, which states RC will on average go down further into the shoe.